How is Focal Length related to Radius of Curvature?
Mirror formula
Definition : The equation relating the object distance (u) the image distance (v) and the mirror focal length (f) is called the mirror formula.
Assumptions made :
- The mirror has a small aperture.
- The object lies close to principal axis of the mirror.
- The incident rays make small angles with the mirror surface or the principal axis.
Linear magnification For spherical mirrors
Definition : The ratio of the size of the image, as formed by reflection from the mirror to the size of the object, is called linear magnification produced by the mirror. It is represented by the symbol m.
Power of mirror
Power of a mirror [in Diopters] = \(\frac { 1 }{ f(in\quad metre) } \)
People also ask
- What is Reflection of Light?
- What is the Law of Reflection of Light?
- What do you mean by Total Internal Reflection?
- Applications of Total Internal Reflection
- Image Reflection by a Plane Mirror
- Which Type of Image is Formed by a Plane Mirror?
- Is an Image formed by Reflection Real or Virtual
- Reflection of Light from Spherical Mirror
- What are Concave and Convex Mirrors?
- How is the Image Formed by a Spherical Mirror?
Focal Length related to Radius of Curvature Example Problems with Solutions
Example 1: An object is placed in front of a plane mirror. If the mirror is moved away from the object through a distance x, by how much distance will the image move?
Solution: Suppose the object O was initially at a distance d from the plane mirror M as shown in fig. The image formed at O’ is at a distance d behind the mirror. Now, the mirror is shifted by a distance x to M’ such that the distance of the object from M’ becomes d + x. The image now formed at O” which is also at a distance d + x from M’.
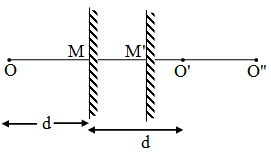So, OM = MO’ = d
OM’ = M’O” = d + x
Thus, OO” = OM’ + M’O” = 2(d + x) …(1)
when OO’ = OM + MO’ = 2d …(2)
∴ O’O” = OO” – OO’
= 2(d + x) – 2d
= 2x
Thus, the image is shifted from O’ to O” by a distance 2x.
Example 2: An insect is at a distance of 1.5m from a plane mirror. Calculate the following?
(i) Distance at which the image of the insect is formed.
(ii) distance between the insect and its image.
Solution:
(i) The distance of insect from the mirror
= 1.5 m
∴ The distance of insect from the mirror is also equal to 1.5 m. The image is formed at 1.5 m behind the mirror.
(ii) The distance between the insect and image
= 1.5 + 1.5 = 3m
Example 3: A concave mirror is made up by cutting a portion of a hollow glass sphere of radius 30 cm. Calculate the focal length of the mirror.
Solution: The radius of curvature of the mirror = 30 cm
Thus, the focal length of the mirror
=\(\frac { 30 cm }{ 2 } \) = 15 cm
Example 4: An object is placed at a distance of 15 cm from a concave mirror of focal length 10 cm. Find the position of the image.
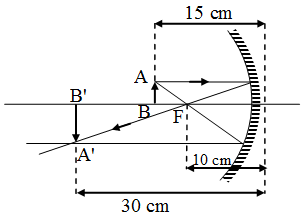Solution:
We have u = –15 cm and f = –10 cm
Using the relation, \(\frac { 1 }{ v } +\frac { 1 }{ u } =\frac { 1 }{ f }\) we get
\(\frac { 1 }{ v } +\frac { 1 }{ -15 } =\frac { 1 }{ -10 } \)
or \(\frac { 1 }{ v } =\frac { 1 }{ 15 } -\frac { 1 }{ 10 } =-\frac { 1 }{ 30 } \)
or v = –30 cm
So the image will be formed 30 cm from the mirror. Since n has a negative sign, the image is formed to the left of the mirror, i.e. in front of the mirror as shown in fig.
Example 5: A 3 cm long object is placed perpendicular to the principal axis of a concave mirror. The distance of the object from the mirror is 15 cm, and its image is formed 30 cm from the mirror on the same side of the mirror as the object . Calculate the height of the image formed.
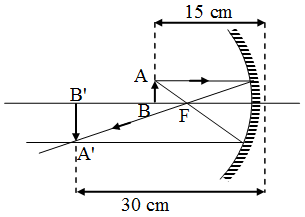
Solution: Here u = –15 cm and n = –30 cm
Size of the object, h = 2 cm
Magnification, \(m=\frac { h’ }{ h } =-\frac { v }{ u } \)
or \(\frac { h’ }{ h } =-\frac { (-30) }{ (-15) } =2 \)
or h’ = – 2 × h = – 2 × 3
= – 6 cm
So the height of the image is 6 cm. The minus sign shows that it is on the lower side of the principal axis, i.e. the image is inverted.
Example 6: A 1.4 cm long object is placed perpendicular to the principal axis of a convex mirror of focal length 15 cm at a distance of 10 cm from it. Calculate the following :
(i) location of the image
(ii) height of the image
(iii) nature of the image
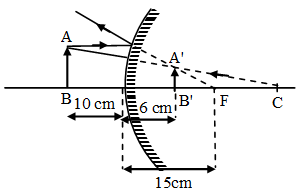
Solution:
(i) For a convex mirror, focal length is positive.
Therefore, f = +15 cm and u = –10 cm
Using the relation, \(\frac { 1 }{ v } +\frac { 1 }{ u } =\frac { 1 }{ f } \), we get
\(\frac { 1 }{ v } +\frac { 1 }{ -10 } =\frac { 1 }{ 15 } \)
or \(\frac { 1 }{ v } =\frac { 1 }{ 15 } +\frac { 1 }{ 10 } =\frac { 5 }{ 30 } =\frac { 1 }{ 6 } \)
or v = 6 cm
Since v is positive, the image is formed to the right of the mirror at a distance 6 cm from it.
(ii) Magnification,
or \(m=\frac { h’ }{ h } =-\frac { v }{ u } \)
\(\frac { h’ }{ h } =\frac { (-6) }{ (-10) } =+0.6 \)
or h’ = + 0.6 × h0 = 0.6 × 1.4
= 0.84 cm.
Thus, the height of the image is 0.84 cm.
(iii) Since h’ is positive, the image will be on the same side of the principal axis as the object.
Hence, the image is virtual, erect and diminished.
Example 7: An object is placed at a distance of 40 cm from a convex mirror of focal length 30 cm. Find the position of image and its nature.
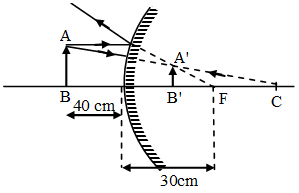
Solution: Here, object distance, u = –40 cm
Focal length of convex mirror, f = +30 cm
Now, using mirror formula, \(\frac { 1 }{ v } +\frac { 1 }{ u } =\frac { 1 }{ f } \) we get
\(\frac { 1 }{ v } +\frac { 1 }{ -40 } =\frac { 1 }{ 30 } \)
or \(\frac { 1 }{ v } =\frac { 1 }{ 40 } +\frac { 1 }{ 30 } =\frac { 7 }{ 120 } \)
or v = \(\frac { 120 }{ 7 } \)
The positive sign shows that the image is formed on the right, i.e. behind the mirror.
Now, magnification,
⇒ \(m=-\frac { v }{ u } =-\frac { 120 }{ 7\times (-40) } =+\frac { 3 }{ 7 } \)
Since, the magnification is positive, the image is erect. Thus, the image is formed 17.1 cm behind the mirror. The image is virtual, erect and diminished.