How To Find The Area Of A Plane Figure

How To Find The Area Of A Plane Figure

The area of the portion of the plane or shape can be defined as the amount of stuff required to cover it.

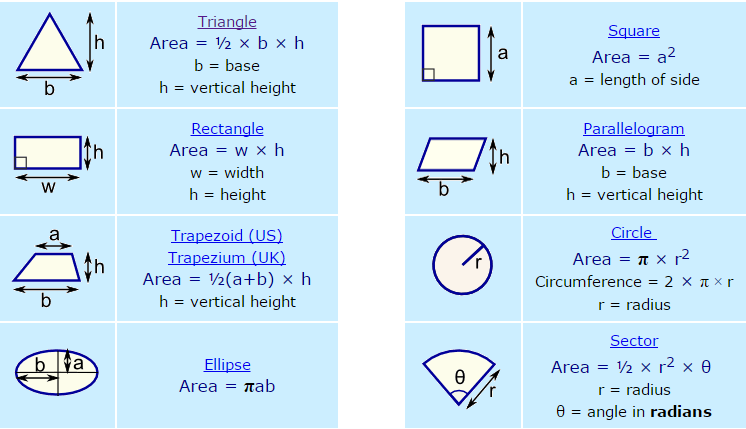
For finding the area of a polygon, we consider the enclosed region of the polygon. Let us consider an illustration to clarify the idea.

A news bulletin board in a class is to be covered with squared colour paper sheets. To know that how many colour paper sheets are required for the said purpose, the best way is to lay the board on the floor and cover it with a coloured paper sheet without any gap as shown in the figure.

Count the number of sheets used for this purpose. Suppose 21 sheets are used to cover the complete board, then, we can measure the region enclosed by a polygon. This measurement is called the area of the polygon.
∴ Area = 21 × Area of 1 sheet
The amount of surface enclosed by a plane figure is called its area. It is measured in square units of lengths.

To find the area of a figure using a graph

We can find the area of regular and irregular figures by using a graph or squared paper. To find the area, first we draw the figure on the graph paper covering as many squares as possible.

For finding the area by using squared paper, we have to follow the following steps:

1. Count the number of complete squares of unit length enclosed by the figure.
2. Count the number of those squares whose more than half parts are enclosed by the figure.
3. Count the number of those squares whose exact half parts are enclosed by the figure and divide the number by 2.
4. Leave those squares whose less than half parts are enclosed by the figure.
5. The total of steps (i), (ii), and (iii) are added to obtain the area of the figure.

∴ Area of the figure = Number of complete squares + Number of squares having more than half parts enclosed + 1/2 (Number of squares having half parts enclosed)

Consider the figures given in figure.
(i) Area of rectangle ABCD
= 8 × Area of one square
= 8 × 1 cm² = 8 cm²
Thus, if the length and breadth of a rectangle are 4 cm and 2 cm respectively, then
Area = 8 cm²
= (4 × 2) sq cm
∴ Area = length × breadth

(ii) Area of ΔPQR = Number of complete square + Number of squares having more than half parts + 1/2 × Number of halved squares
Number of complete squares enclosed in the figure = 2
Number of squares whose more than half parts are enclosed = 4
Neglect the number of squares whose less than half part is enclosed.
Area of ΔPQR = 2 + 4 + 1/2 × 0
= 6 squares
= 6 × 1 cm²
= 6 cm²
Thus, if the base and height of the triangle are 4 cm and 3 cm respectively, then
Area = 6 cm²
= 1/2 × 4 × 3
= 1/2 × base × height

(iii) Area of square WXYZ
= 9 × Area of one square
= 9 × 1 cm²
= 9 cm²
Thus, if the side of the square is 3 cm, then
Area = 9 cm²
= (3 × 3) cm²
= side × side

(iv) Similarly, area of figure ABCDEFGH
= 7 × Area of one square
= 7 × 1 cm²
= 7 cm²

The Area Of Plane Figures Example Problems with Solutions

Example 1: Find the area of a square whose side is 8 cm.
Solution: Area of square
= side × side
= 8 cm × 8 cm
= 64 cm²

Example 2: Find the area of a rectangle whose length is 21 cm and breadth is 5 cm.
Solution: Area of a rectangle
= length × breadth
= 21 cm × 5 cm
= 105 cm²

Example 3: The side of a square plot of land is 30 m. Find the cost of levelling the plot, if the rate is Rs 2 per square metre.
Solution: Area of square
= side × side
= 30 m × 30 m
= 900 m²
Levelling cost of 1 m²
= Rs. 2
Levelling cost of 900 m²
= Rs. (2× 900)
= Rs. 1800

Example 4: Find the area of the given figure.

Solution: Area of the rectangle DEFG = l × b
= 7 cm × 3 cm = 21 cm²
Area of the rectangle ABCG  l × b
= 7 cm × 3 cm = 21 cm²
Area of the complete figure
= Area of rectangle DEFG
= 21 cm² + 21 cm²
= 42 cm²

Example 5: How many tiles, each measuring 2 m by 1 m, are required to cover a rectangular hall 12 m long and 8 m broad? Find the cost of tiles at Rs. 25 per tile.
Solution: Length of a tile = 2 m
Breadth of a tile = 1 m
∴ Area of each tile = 2 m × 1 m
= 2 m²
Length of rectangular hall = 12 m
Breadth of rectangular hall = 8 m
∴ Area of the hall = 12 m × 8 m
= 96 m²
Hence, number of tiles required
= Area of the hail/Area of one tile
= 96/2 = 48
∴ Cost of tiles 48 × 25
= Rs. 1200