What is Empirical and Molecular Formula?
Empirical formulae and molecular formulae:
- A compound can be represented by two types of chemical formulae.
(a) Empirical formula
(b) Molecular formula
- A compound can be represented by two types of chemical formulae.
- Empirical formula of a compound gives the simplest whole number ratio of atoms of each element present in the compound.
- Molecular formula of a compound gives the actual number of atoms of each element present in one molecule of the compound.
- (a) For example, C6H12O6 is the molecular
formula for glucose. Thus, each molecule of glucose consists of 6 carbon atoms, 12 hydrogen atoms and 6 oxygen atoms.
(b) The simplest ratio of carbon to hydrogen to oxygen atoms in the molecule is 1:2:1. Therefore, the empirical formula of glucose is CH2O. - Table below shows the molecular and empirical formulae of some compounds.
Compound Molecular formula Simplest ratio of atoms of elements Empirical formula Water H2O H:O = 2:1 H2O Ethene C2H4 C:H = 1:2 CH2 Benzene C6H6 C:H = 1:1 CH Vitamin C C6H806 C:H:O = 3:4:3 C3H4O3. - Similarities between empirical and molecular formulae:
- Both show the elements present in a compound.
- Both show the ratio of atoms of each element in a compound.
- Differences between empirical and molecular formulae:
Empirical formula Molecular formula Shows the simplest ratio of atoms of each element in a compound. Shows the actual number of atoms of each element in a molecule of the compound.
People also ask
- What is the Relative Atomic Mass and Relative Molecular Mass of an Element?
- What is One Mole and How many Particles are in a Mole?
- How do you Calculate the Molar Mass of a Substance?
- What is the Molar Volume of a Gas at STP?
- How do you know the Order of Elements in a Chemical Formula
- How do you Write a Chemical Equation?
Determining empirical formulae
- The empirical formula of a compound is determined experimentally by finding the simplest ratio of moles of its elements.
- The following shows the steps in determining the empirical formula of a compound.
- Find the mass of each element in the compound.
- Convert the masses to numbers of moles of atoms.
- Find the simplest ratio of moles of the elements.
Empirical formula Problems with Solutions
1. 1.08 g of aluminium combines chemically with 0. 96 g of oxygen to form an oxide. What is the empirical formula of the oxide? [Relative atomic mass: O, 16; Al, 27]
Solution:
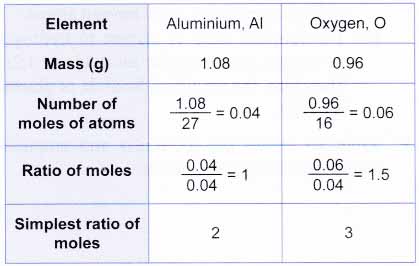 Since 2 moles of aluminium atoms combine with 3 moles of oxygen atoms, the empirical formula of the oxide is Al2O3.
Since 2 moles of aluminium atoms combine with 3 moles of oxygen atoms, the empirical formula of the oxide is Al2O3.
2. Copper(II) iodide contains 20.13% copper by mass. Find its empirical formula. [Relative atomic mass: Cu, 64; I, 127]
Solution:
Based on its percentage composition, we know that every 100 g of copper(II) iodide contains 20.13 g of copper. So by taking 100 g of the compound:
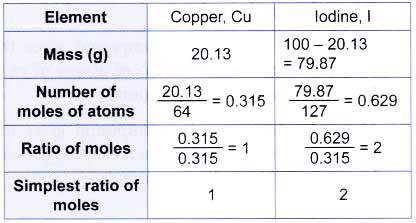 1 mole of copper atoms combine with 2 moles of iodine atoms. Therefore, the empirical formula of copper(II) iodide is CuI2.
1 mole of copper atoms combine with 2 moles of iodine atoms. Therefore, the empirical formula of copper(II) iodide is CuI2.
How do you find the empirical formula from percentages?
Example: Phosphoric acid has the percentage composition as follows.
H, 3.06%; P, 31.63%; O, 65.31%
What is the empirical formula of the acid? [Relative atomic mass: H, 1; O, 16; P, 31]
Solution:
From the percentage composition, every 100 g of the compound contains 3.06 g of hydrogen, 31.63 g of phosphorus and 65.31 g of oxygen. So, by taking 100 g of the compound:
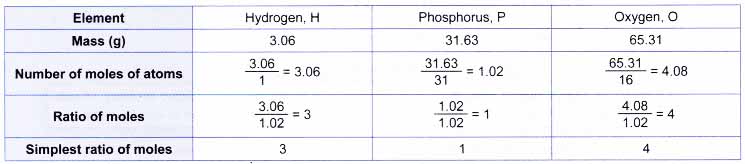 3 moles of hydrogen atoms combine with 1 mole of phosphorus atoms and 4 moles of oxygen atoms. Therefore, the empirical formula of the acid is H3PO4.
3 moles of hydrogen atoms combine with 1 mole of phosphorus atoms and 4 moles of oxygen atoms. Therefore, the empirical formula of the acid is H3PO4.
Determination of the Empirical Formula of Copper Oxide
Aim: To determine the empirical formula of copper(II) oxide.
Materials: 2 mol dm3 sulphuric acid, 1 mol dm-3 copper(II) sulphate solution, zinc granules, copper(II) oxide, anhydrous calcium chloride and wooden splinter.
Apparatus: Combustion tube with a small hole at the end, Bunsen burner, flat-bottomed flask, thistle funnel, stoppers, glass tube, retort stand and clamp, balance, U-tube, spatula and porcelain dish.
Procedure:
- The mass of the combustion tube with the porcelain dish in it is weighed.
- A spatulaful of copper(II) oxide is added to the porcelain dish. The tube is weighed again.
- The apparatus is set up as shown in Figure.
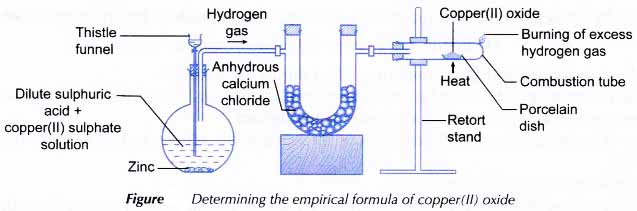
- Hydrogen gas is allowed to flow into the set of apparatus for 5 to 10 minutes to remove all the air in the tube.
- To determine whether all the air has been removed from the tube, the gas that comes out from the small hole is collected in a test tube. Then, the gas is tested with a lighted wooden splinter. If the gas burns quietly without a ‘pop’ sound, then all the air has been totally removed from the combustion tube.
- The excess hydrogen gas that flows out from the small hole of the combustion tube is burnt and the copper(II) oxide is heated strongly.
- The flame is turned off when the copper(II) oxide turns completely brown.
- The flow of hydrogen gas is continued until the set of apparatus cools down to room temperature.
- The mass of the combustion tube with its content is weighed again.
- The heating, cooling and weighing are repeated until a constant mass is obtained. The constant mass is recorded.
Results:
| Description | Mass (g) |
| Combustion tube + porcelain dish | x |
| Combustion tube + porcelain dish + copper(II) oxide | y |
| Combustion tube + porcelain dish + copper | z |
| Copper | z – x |
| Oxygen | y-z |
Calculation:
[Relative atomic mass: O, 16; Cu, 64]
| Element | Copper, Cu | Oxygen, O |
| Mass (g) | z-x | y-z |
| Number of moles of atoms | (z-x)/64 | (y-z)/16 |
| Simplest ratio of moles | m | n |
Based on the calculation, m moles of copper atoms combine with n moles of oxygen atoms. Therefore, the empirical formula of copper(II) oxide is CumOn.
 Discussion:
Discussion:
- The function of anhydrous calcium chloride is to dry the hydrogen gas.
- Copper(II) oxide is black in colour. It reacts with hydrogen gas to produce brown copper metal.
Hydrogen gas + copper(II) oxide → copper + water - Below are the precautions and safety measures taken during the activity.
(a) Air in the combustion tube must be totally removed before step 6 is carried out. Otherwise, a mixture of hydrogen gas and air will cause an explosion when lighted.
(b) The flow of hydrogen gas must be continuous throughout this activity so that air does not enter the tube. Otherwise,
(i) an explosion may occur, and
(ii) the hot copper produced will react with the oxygen in the air to form copper(II) oxide again.
(c) Heating, cooling and weighing are repeated until a constant mass is obtained to ensure that all copper(II) oxide has changed into copper. - This method can also be used to determine the empirical formulae of oxides of other low reactivity metals such as tin(II) oxide and lead(II) oxide.
- This method cannot be replaced by heating copper(II) oxide with reactive metals such as magnesium or calcium. Both the reactants and products are solids and thus the individual mass of copper and oxygen cannot be determined at all.
Conclusion:
The empirical formula of copper(II) oxide is CuO where m = 1 and n = 1.
Determination of the Empirical Formula of Magnesium Oxide
Aim: To determine the empirical formula of magnesium oxide.
Materials: 10 cm magnesium ribbon and sandpaper.
Apparatus: Crucible with lid, tongs, Bunsen burner, tripod stand, pipe-clay triangle and balance.
Procedure:
- A crucible and its lid are weighed.
- A 10 cm length of magnesium ribbon is cleaned with sandpaper to remove the oxide layer on its surface.
- The ribbon is coiled loosely and is placed in the crucible. The crucible with its lid and content are weighed.
- The apparatus is set up as shown in Figure.
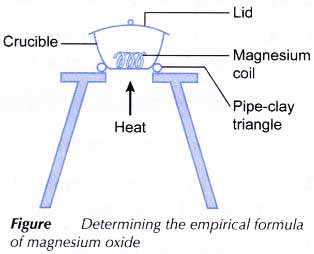
- The crucible is heated strongly without its lid.
- When the magnesium starts to burn, the crucible is covered with its lid.
- Using a pair of tongs, the lid is carefully raised a little at intervals. When the burning is complete, the lid is removed and the crucible is heated strongly for 1 to 2 minutes.
- The crucible is allowed to cool to room temperature with its lid still on.
- The crucible with its lid and content are weighed again.
- The processes of heating, cooling and weighing are repeated until a constant mass is obtained. The constant mass obtained is recorded.
Results:
| Description | Mass (g) |
| Crucible + lid | x |
| Crucible + lid + magnesium | y |
| Crucible + lid + magnesium oxide | z |
| Magnesium | y-x |
| Oxygen | z-y |
Calculation:
[Relative atomic mass: O, 16; Mg, 24]
| Element | Magnesium, Mg | Oxygen, O |
| Mass (g) | y-x | z-y |
| Number of moles of atoms | (y-x)/24 | (z-y)/16 |
| Simplest ratio of moles | r | s |
Based on the calculation, r moles of magnesium atoms combine with s moles of oxygen atoms. Therefore, the empirical formula of magnesium oxide is MgrOs.
Discussion:
- Magnesium reacts with oxygen in the air to form white fumes, magnesium oxide.
Magnesium + oxygen → magnesium oxide - Below are the precautions taken in this activity.
(a) The lid is removed at intervals to allow oxygen to enter the crucible and react with magnesium.
(b) The crucible is then quickly covered with its lid to prevent the white fumes of magnesium oxide from escaping. This would affect the accuracy of the mass obtained.
(c) Heating, cooling and weighing are repeated until a constant mass is obtained to ensure that the magnesium ribbon reacts completely to form magnesium oxide. - This method can also be used to determine the empirical formulae of oxides of other high reactivity metals such as calcium oxide, aluminium oxide and zinc oxide.
Conclusion:
The empirical formula of magnesium oxide is MgO where r = 1 and s = 1.
Determining molecular formulae
- Actually, the molecular formula of a compound is a multiple of its empirical formula.
Molecular formula = (empirical formula)n whereby n is a positive integer. - Table below shows the molecular and empirical formulae of some compounds.
- The molecular formulae of some compounds are similar to the empirical formulae of the compounds. Note that the value of n is 1. However, some compounds do not have similar molecular and empirical formulae.
Table below Empirical and molecular formulae of some compoundsCompound Empirical formula n Molecular formula Water H2O 1 (H2O)1 = H2O Ethane CH3 2 (CH3)2 = C2H6 Propene CH2 3 (CH2)3 = C3H6 Glucose CH2O 6 (CH2O)6 = C6H12O6 Ethanoic acid CH2O 2 (CH2O)2 = C2H4O2 or CH3COOH - Molecular formula of a compound can be determined if we know the following data:
(a) Its empirical formula
(b) Its relative molecular mass or molar mass - If the empirical formula is not given, first, you need to determine it.
Molecular formula Problems with Solutions
1. A carbon compound has an empirical formula of CH, and a relative molecular mass of 70. Find the molecular formula of the compound. [Relative atomic mass: H, 1; C, 12]
Solution:
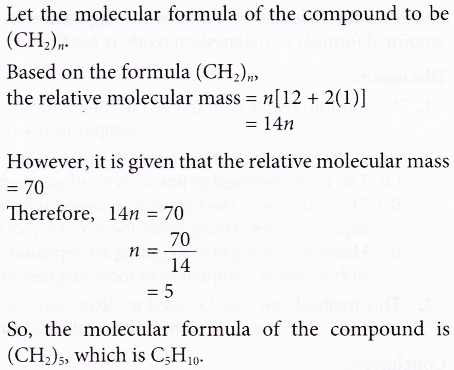
2. 8.5 g of hydrogen peroxide contains 0.5 g of hydrogen. If the molar mass of hydrogen peroxide is 34 g mol-1, find its molecular formula. [Relative atomic mass: H, 1; O,16]
Solution:
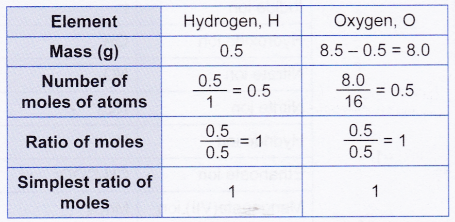 1 mole of hydrogen atoms combines with 1 mole of oxygen atoms.
1 mole of hydrogen atoms combines with 1 mole of oxygen atoms.
Therefore, the empirical formula of hydrogen peroxide is HO.
Let its molecular formula to be (HO)n.
Based on the formula (HO)n, its relative molecular mass = n[ 1 + 16] = 17n
However, it is given that the molar mass of hydrogen peroxide is 34 g mol-1.
This means that its relative molecular mass = 34
Therefore, 17n = 34
n = 34/17
n = 2
So, the molecular formula of hydrogen peroxide is (HO)2, which is H2O2.
Calculations involving empirical and molecular formulae
- Both empirical and molecular formulae enable us to solve numerical problems concerning the composition of compounds.
- we can also calculate the percentage composition by the mass of compounds using their chemical formulae.

1. What is the mass of copper needed to combine completely with 0.5 mole of chlorine atoms to form copper(II) chloride, CuCl2? [Relative atomic mass: Cu, 64]
Solution:
Based on the formula CuCl2, 2 moles of chlorine atoms combine with 1 mole of copper atoms.
Therefore, 0.5 mole of chlorine atoms combines 0.5/2 with mole of copper atoms, that is 0.25 mole of copper atoms.
Mass of copper needed
= number of moles of copper atoms × molar mass of copper
= 0.25 × 64
= 16 g
2. 2.07 g of element Z reacts with bromine to form 3.67 g of a compound with the empirical formula of ZBr2. Find the relative atomic mass of element Z. [Relative atomic mass: Br, 80]
Solution:
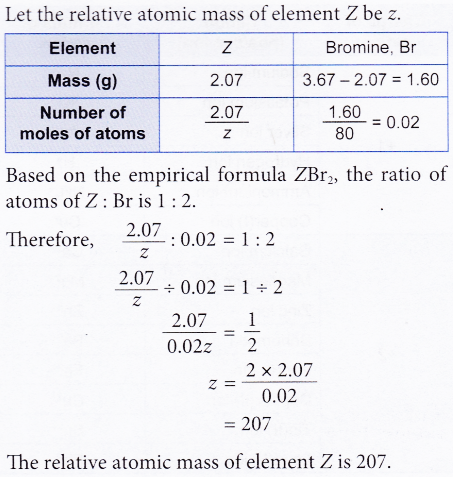
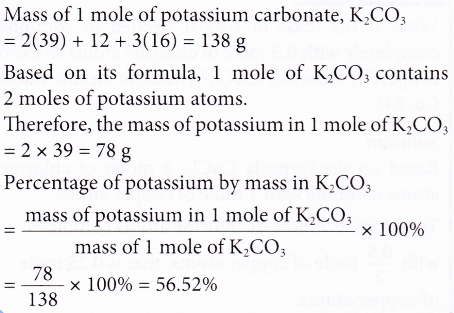
3. Calculate the percentage of potassium by mass in potassium carbonate. [Relative atomic mass: C, 12; O, 16; K, 39]
Solution: