Direct Variation
Examine the graph at the right:
The linear equation graph at the right shows that as the x value increases, so does the y value increase for the coordinates that lie on this line .
For instance, if x = 2, y = 4.
If x = 6 (multiplied by 3),
then y = 12 (also multiplied by 3).
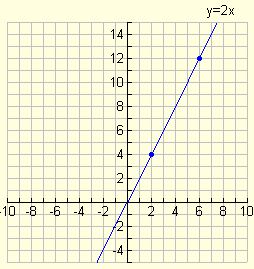
This is a graph of direct variation. If the value of x is increased, then y increases as well.
Both variables change in the same manner.
If x decreases, so does the value of y. We say that y varies directly as the value of x.
A direct variation between 2 variables, y and x, is a relationship that is expressed as:
y=kx.
where the variable k is called the constant of proportionality.
In most problems, the k value needs to be found using the first set of data given.
Examples:
Find the Constant, k:
The power, P, of a gear varies directly with the
radius, r, of a gear. Find the constant of proportionality
if P = 300 when r = 50.
Start with the formula: p=kr
Substitute the values: 300 = 50k
then solve for k: k=6
Typical Direct Variation Problem:
In a factory, the profit, P, varies directly with
the inventory, I.
If P = 100 when I = 20, find P when I = 50.
It will be necessary to use the “first” set of data to find the value for the constant, k.
As in most variation problems, it is necessary to do this problem in three steps:
1. Set up the formula.
p =lk
2. Find the missing constant, k, using the first set of data given.
100=20k or k =5
3. Using the formula and constant, k, find the missing value in the problem.
p=lk
p=50.5
p=250
Joint Variation Problem:
Sometimes more than one variable is involved in a direct variation problem. In these cases, the problem is referred to as a joint variation. The formula remains the same, with the additional variables included in the product.
For example: If P varies jointly as the values of R and S, then the formula will be:
P= K RS
Joint Variation Example:
Variable M varies jointly as the values of p and q.
If M = 88 when p = 4 and q = .4,
find M when p = 8 and q = 1.2.
Use the same three process steps:
1. Set up the formula.
M= kpq
2. Find the missing constant
of proportionality, k.
M= kpq
88= 4(0.4)k
k=55
3. Using the formula and constant, k, find the new value in the problem.
M= kpq
M= 55 (8)(1.2)
M=528
