Conic Sections
Definition:
The curves obtained by intersection of a plane and a double cone in different orientation are called conic section.
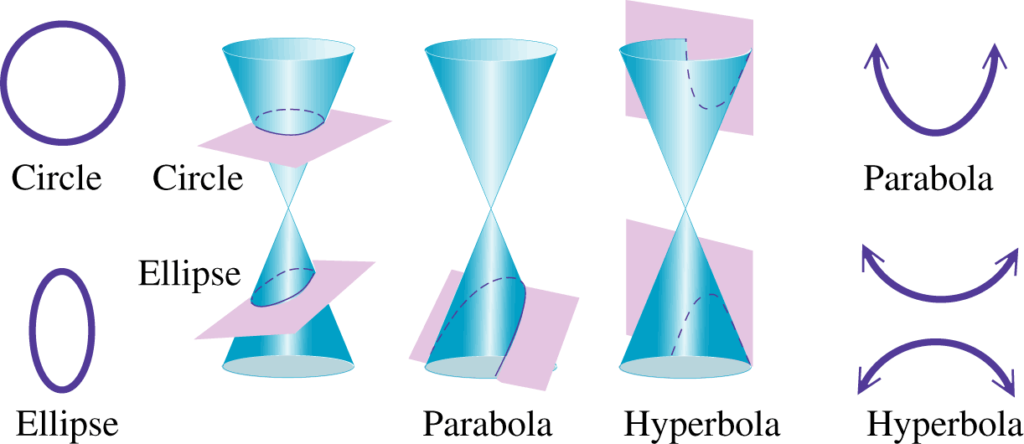
Definitions of various important terms:
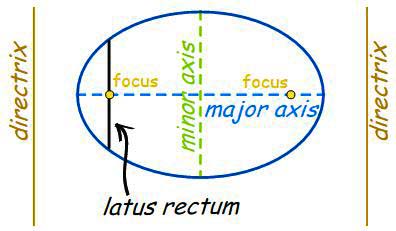
- Focus: The fixed point is called the focus of the conic-section.
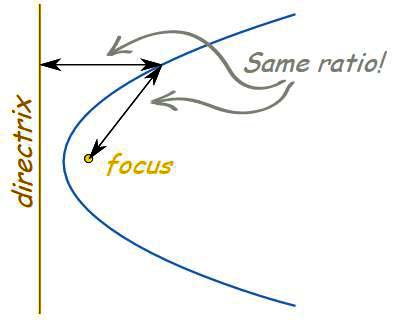
- Directrix: The fixed straight line is called the directrix of the conic section.
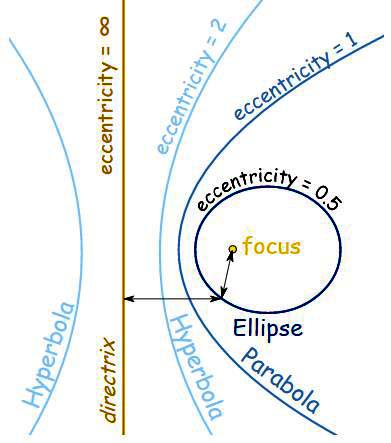
- Eccentricity: The constant ratio is called the eccentricity of the conic section and is denoted by e.
- Axis: The straight line passing through the focus and perpendicular to the directrix is called the axis of the conic section. A conic is always symmetric about its axis.
- Vertex: The points of intersection of the conic section and the axis are called vertices of conic section.
- Centre: The point which bisects every chord of the conic passing through it, is called the centre of conic.
- Latus-rectum: The latus-rectum of a conic is the chord passing through the focus and perpendicular to the axis.
- Double ordinate: The double ordinate of a conic is a chord perpendicular to the axis.
- Focal chord: A chord passing through the focus of the conic is called a focal chord.
- Focal distance: The distance of any point on the conic from the focus is called the focal distance of the point.
General equation of a conic section when its focus, directrix and eccentricity are given:
Let S(α, β) be the focus, Ax + By + C = 0 be the directrix and e be the eccentricity of a conic.
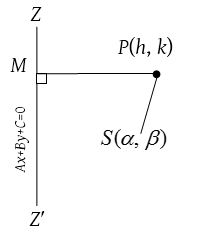Let P(h, k) be any point on the conic. Let PM be the perpendicular from P, on the directrix. Then by definition,
SP = ePM ⇒ SP2 = e2PM2
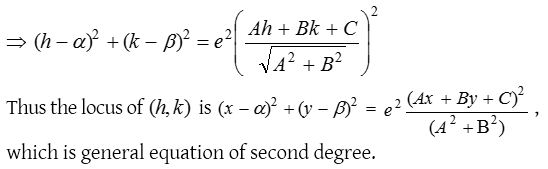
Recognisation of conics
The equation of conics is represented by the general equation of second degree ax2 + 2hxy + by2 + 2gx + 2fy + c = 0 ……(i)
and discriminant of above equation is represented by ∆, where ∆ = abc + 2fgh – af2 – bg2 – ch2
Case I: When ∆ = 0.
In this case equation (i) represents the degenerate conic whose nature is given in the following table.
| S. No. | Condition | Nature of conic |
| 1. | ∆ = 0 and ab – h2 = 0 | A pair of coincident straight lines |
| 2. | ∆ = 0 and ab – h2 < 0 | A pair of intersecting straight lines |
| 3. | ∆ = 0 and ab – h2 > 0 | A point |
Case II: When ∆ ≠ 0.
In this case equation (i) represents the non-degenerate conic whose nature is given in the following table.
| S. No. | Condition | Nature of conic |
| 1. | ∆ ≠ 0, h = 0, a = b, e = 0 | A circle |
| 2. | ∆ ≠ 0, ab – h2 = 0, e = 1 | A parabola |
| 3. | ∆ ≠ 0, ab – h2 > 0, e < 0 | An ellipse |
| 4. | ∆ ≠ 0, ab – h2 < 0, e > 0 | A hyperbola |
| 5. | ∆ ≠ 0, ab – h2 < 0, a + b = 0, e = √2 | A rectangular hyperbola |