CBSE Sample Papers for Class 12 Maths Paper 3 are part of CBSE Sample Papers for Class 12 Maths. Here we have given CBSE Sample Papers for Class 12 Maths Paper 3.
CBSE Sample Papers for Class 12 Maths Paper 3
| Board | CBSE |
| Class | XII |
| Subject | Maths |
| Sample Paper Set | Paper 3 |
| Category | CBSE Sample Papers |
Students who are going to appear for CBSE Class 12 Examinations are advised to practice the CBSE sample papers given here which is designed as per the latest Syllabus and marking scheme as prescribed by the CBSE is given here. Paper 3 of Solved CBSE Sample Paper for Class 12 Maths is given below with free PDF download solutions.
Time: 3 Hours
Maximum Marks: 100
General Instructions:
- All questions are compulsory.
- Questions 1-4 in section A are very short answer type questions carrying 1 mark each.
- Questions 5-12 in section B are short answer type questions carrying 2 marks each.
- Questions 13-23 in section C are long answer I type questions carrying 4 marks each.
- Questions 24-29 in section D are long answer II type questions carrying 6 marks each.
SECTION A
Question 1.
Write the direction cosines of the line equally inclined to the three coordinate axes.
Question 2.
Evaluate ∫π2−π2(sin5x+x3−2x)dx
Question 3.
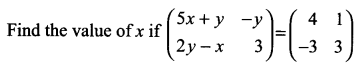
Question 4.
Prove that f(x) = x3 – 3x2 + 3x – 100 is increasing on R.
SECTION B
Question 5.
Prove that the inverse of a square matrix, if it exists, is unique.
Question 6.
An aeroplane can carry a maximum of 250 passengers. A profit of ₹ 500 is made on each first class ticket and a profit of ₹ 350 of each economy class ticket. The airline reserves atleast 25 seats for first class. However, atleast 3 times as many passengers prefer to travel by economy class than first class. Form an L.P.P to determine how many tickets of each type must be added in order to maximize profit for the airlines.
Question 7.
Evaluate ∫20(x−[x])dx
Question 8.
Evaluate P(A ∪ B), if 2P(A) = P(B) = 113 and P(A|B) = 25
Question 9.
The length x of a rectangle is decreasing at the rate of 5 cm/minute and the width y is increasing at the rate of 4 cm/minute. When x = 8 cm and y = 6 cm, find the rate of change of the area of the rectangle.
Question 10.
If y = cos-1x, find d2ydx2 in terms of y alone.
Question 11.
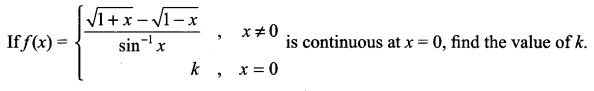
Question 12.
Show that the lines x = ay + b, z = cy + d and x = a’y + b’, z = c’y + d’ are perpendicular if aa’ + cc’ + 1 = 0.
SECTION C
Question 13.
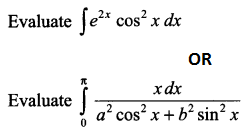
Question 14.
Solve the following differential equation
xdydx=y−xtan(yx)
Question 15.
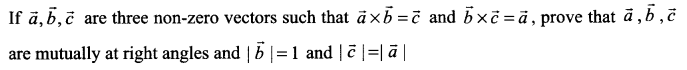
Question 16.
In a hurdle race, a player has to cross 10 hurdles. The probability that he will clear each hurdle is 5/6. What is the probability that he will knock down fewer than 2 hurdles? How can a teacher help the student if he feel same hurdle in study?
OR
In a group of 400 people, 160 are smokers and non-vegetarian, 100 are smokers and vegetarians and the remaining are non-smokers and vegetarians. The probabilities of getting a special chest disease are 35%, 20% and 10% respectively. A person is choosen from the group at random and is found to be suffering from the disease. What is the probability that the selected person is a smoker and non-vegetarian? What value is reflected in this question?
Question 17.
If cos-1x – cos-1 y2 = α, then prove that 4x2 – 4xy cos α + y2 = 4 sin2α.
Question 18.
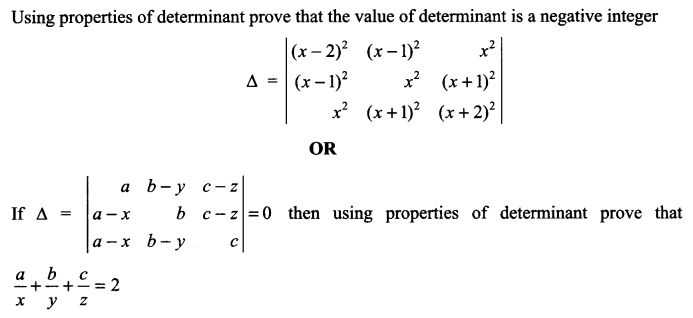
Question 19.
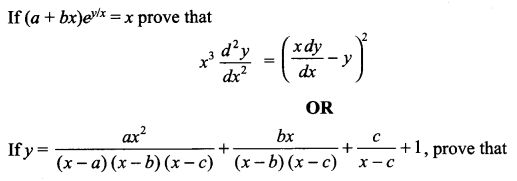
Question 20.
An urn contains 4 white and 3 red balls. Find the probability distribution of the number of red,balls in a random draw of three balls. Also find mean of the distribution.
Question 21.
Solve the following L.P.P.
Minimize and Maximize Z = x + 2y
Subject to constraints x + 2y ≥ 100
2x – y ≤ 0
2x + y ≤ 200
x, y ≥ 0
Question 22.
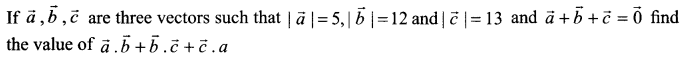
Question 23.
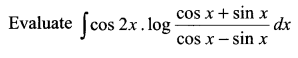
SECTION D
Question 24.
Find the area of the region bounded by the curve y = x2 + 2, y = x, x = 0 and x = 3.
OR
Find the area of the region common to the circle x2 + y2 = 16 and the parabola y2 = 6x
Question 25.
Find the particular solution of the differential equation (x – y) (dx + dy) = dx – dy given that y = -1 and x = 0.
Question 26.
Find the shortest distance between the lines
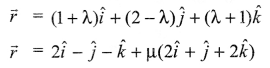
OR
A variable plane which remains at a constant distance 3p from the origin cuts the coordinate axes at A, B and C. Show that the locus of the centroid of the triangle ABC is x-2 + y-2 + z-2 = p-2
Question 27.
Let f : X → Y be an invertible function. Show that the inverse of f-1 is f means (f-1)-1 = f
Question 28.
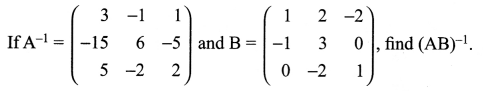
Question 29.
Find the area of the greatest rectangle that can be inscribed in the ellipse x2a2+y2b2=1
Solutions
Solution 1.
Direction cosines of a line equally inclined with the three coordinate axis are ±1√3 , ±1√3 , ±1√3.
Solution 2.
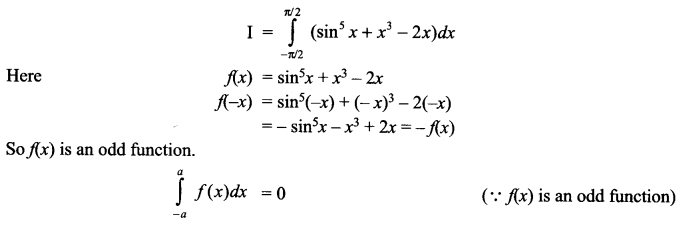
Solution 3.
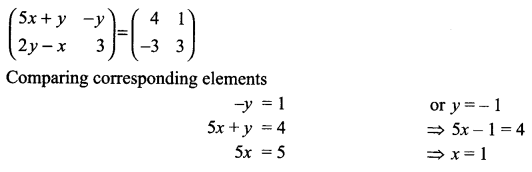
Solution 4.
f(x) = x3 – 3x2 + 3x – 100
f'(x) = 3x2 – 6x + 3 = 3(x2 – 2x + 1) = 3 (x – 1)2
For all values of x ∈ R, f'(x) ≥ 0, so f(x) is increasing on R.
Solution 5.
Let A be a square matrix. If possible let B and C are its two inverses.
As B is the inverse of A
AB = BA = I …(1)
As C is the inverse of A
AC = CA = I …(2)
B = BI = B(AC) = (BA)C = IC = C
B = C
Hence the inverse of A is unique.
Solution 6.
Let the number of first class tickets sold = x
Number of economy class tickets sold = y
Objective function is maximize profit Z = 500x + 350y
Subject to constraints are
x ≥ 25
y ≥ 3x
x + y ≤ 250
x, y ≥ 0
Solution 7.
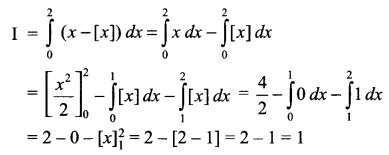
Solution 8.
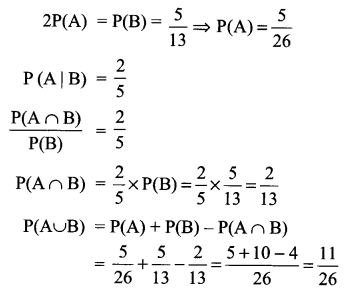
Solution 9.
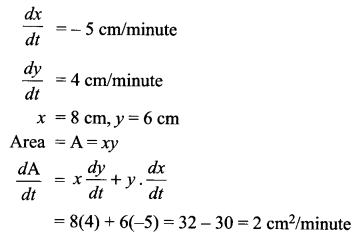
Solution 10.
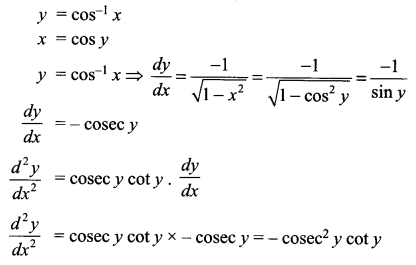
Solution 11.
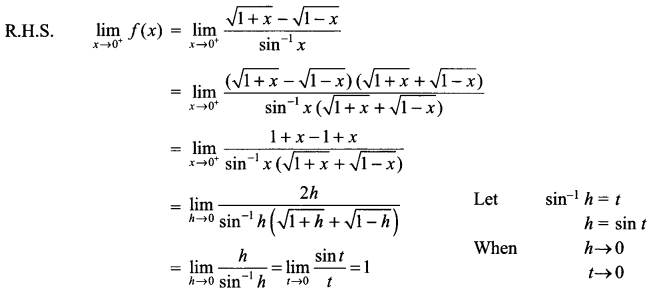
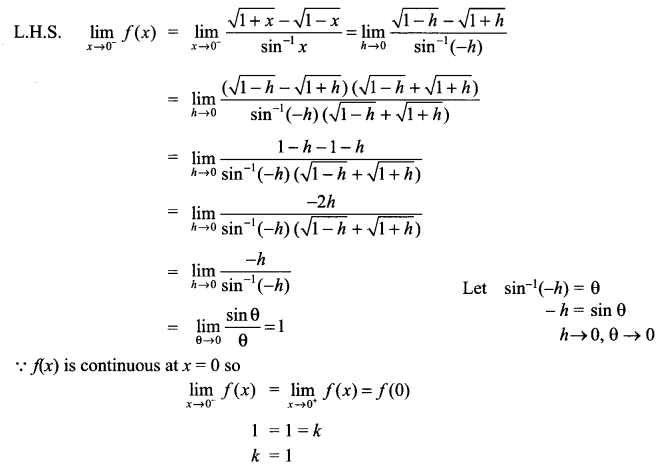
Solution 12.
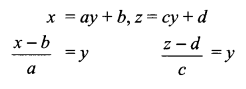
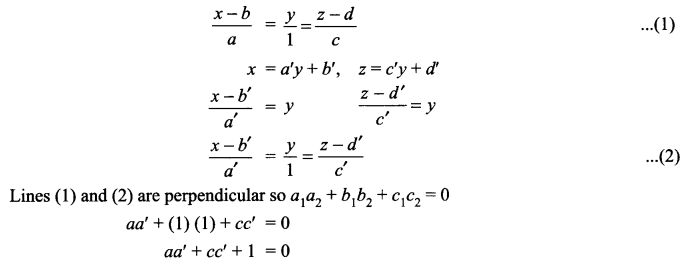
Solution 13.
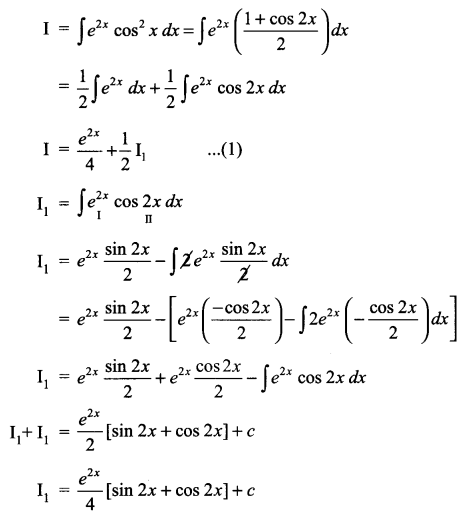
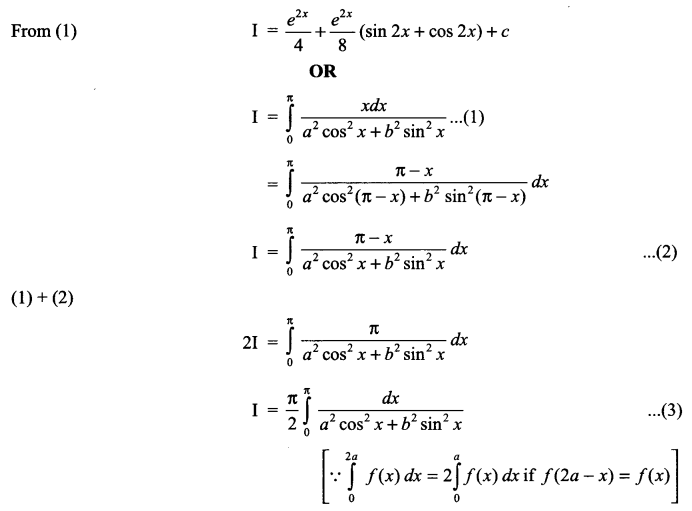
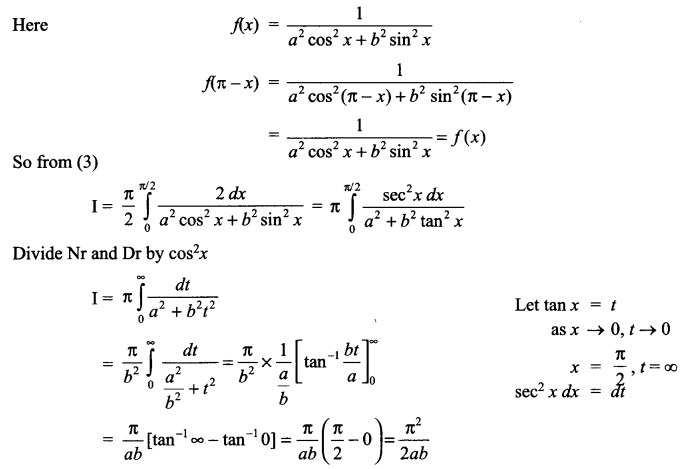
Solution 14.
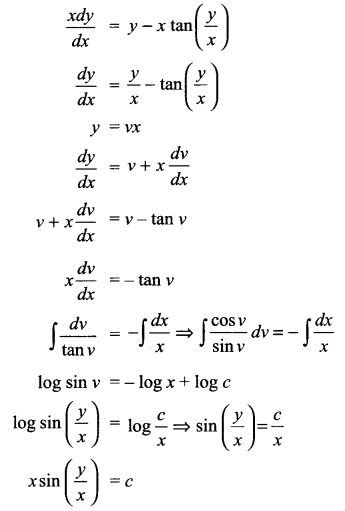
Solution 15.
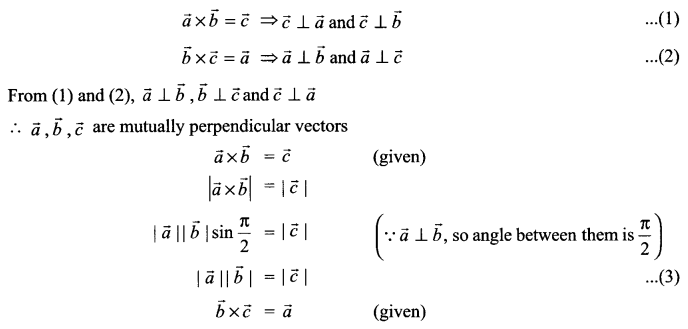
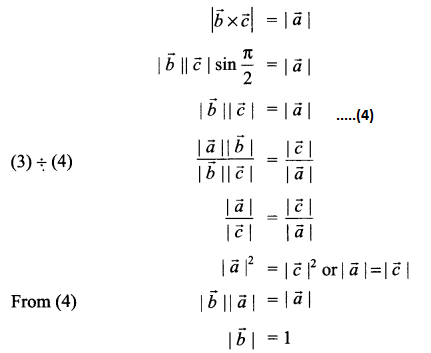
Solution 16.
Let p is the probability that he will knocking down a hurdle.
q – he will clear the hurdle
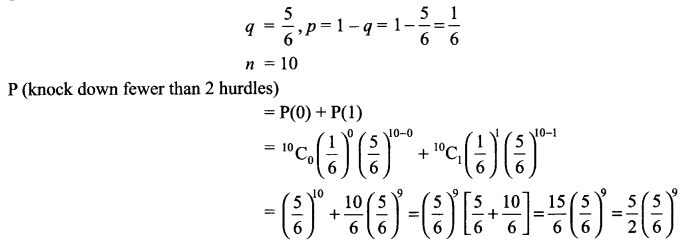
If student feel hurdle in the study, teacher can motivate the students by solving their problems.
OR
Total people in group = 400
E1: Number of people who are smokers and non-vegetarian = 160
E2: Number of people who are smokers and vegetarian = 100
E3: Number of people who are non-smokers and vegetarian = 400 – 160 – 100 = 140
A: Person choosen is found to be suffering from the disease.
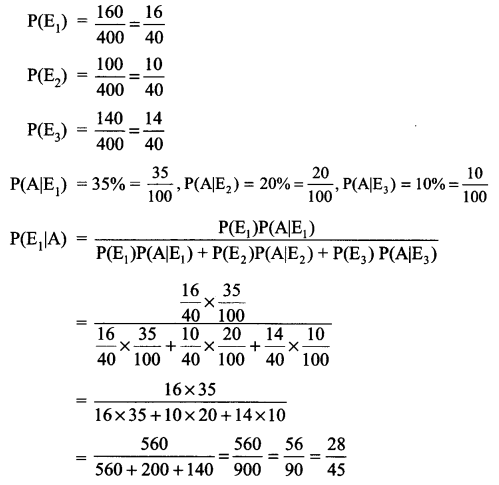
Smoking and non-vegetarian food are not good for health.
Solution 17.
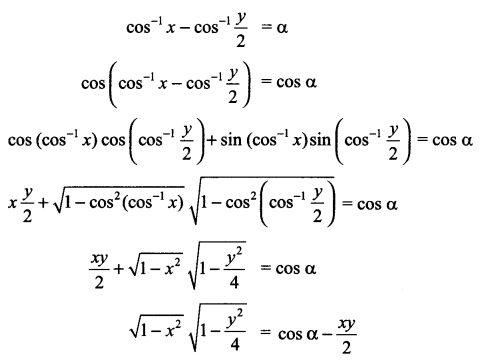
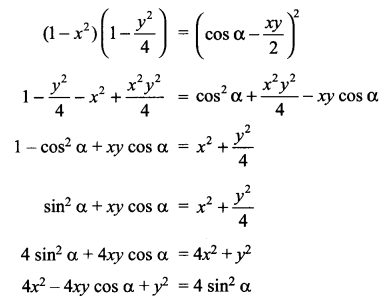
Solution 18.
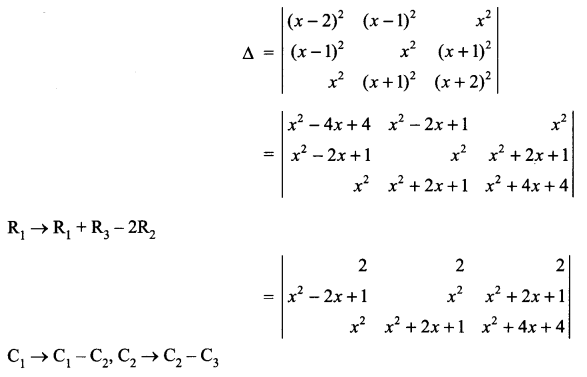
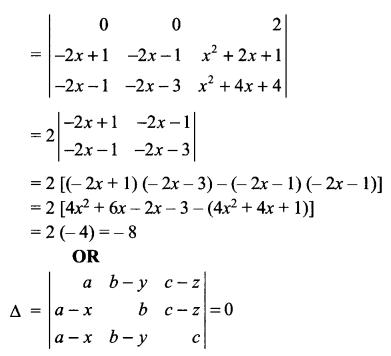
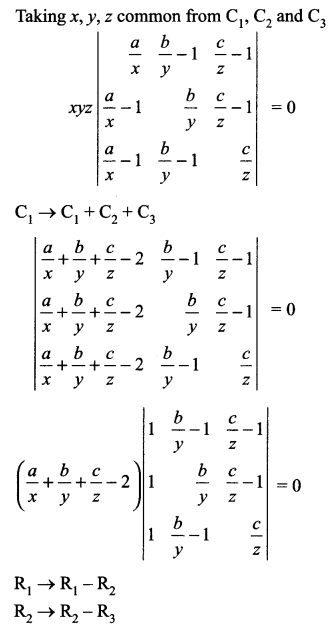
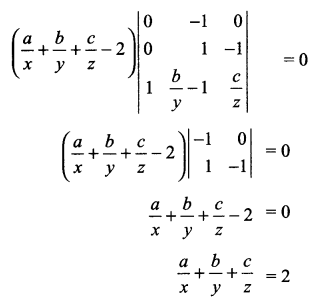
Solution 19.
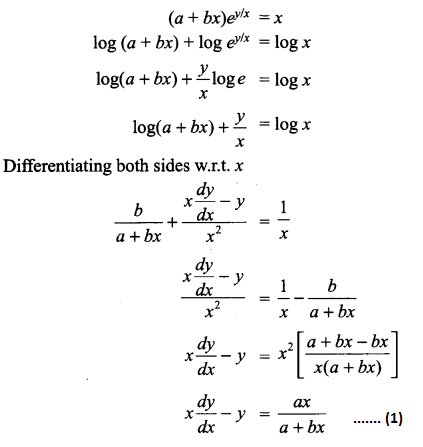

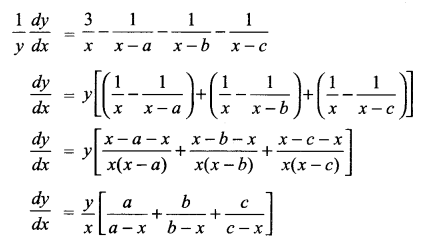
Solution 20.
White balls = 4
Red balls = 3
Total balls = 7
3 balls are drawn at random with replacement.
Let x: denote the number of red balls drawn.
Possible values of x are 0, 1, 2, 3
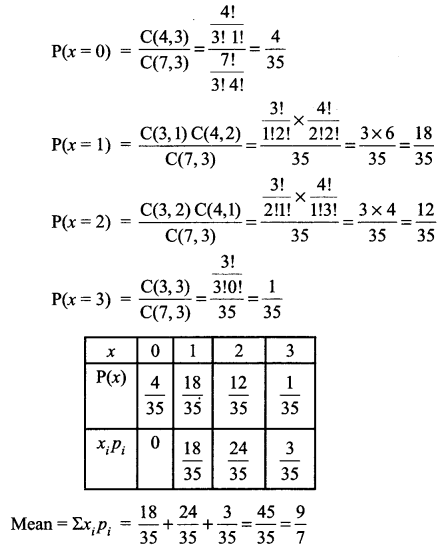
Solution 21.
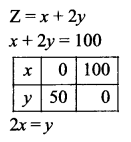
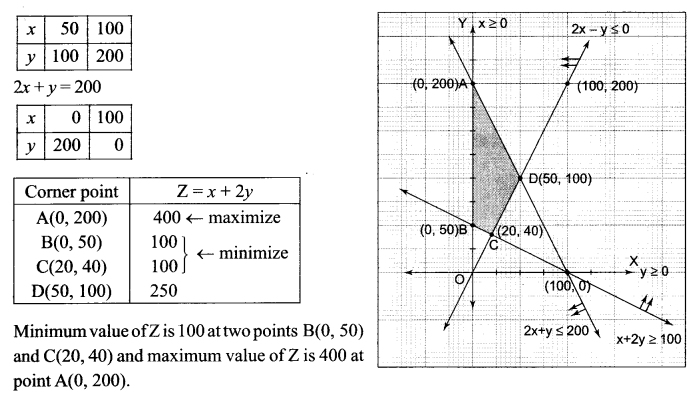
Solution 22.
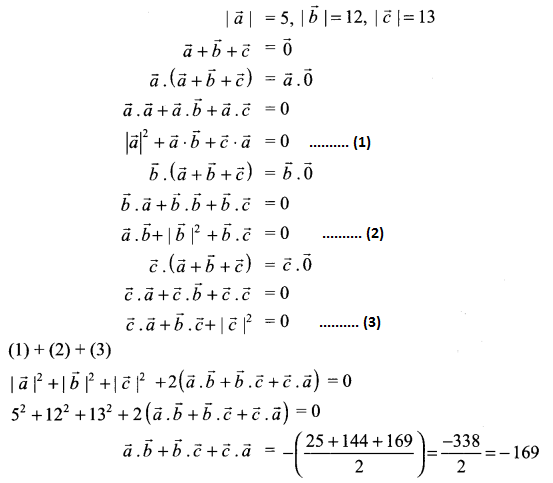
Solution 23.
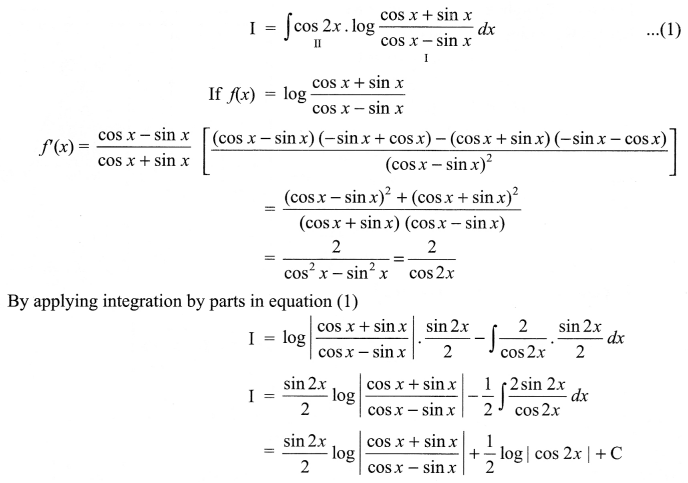
Solution 24.
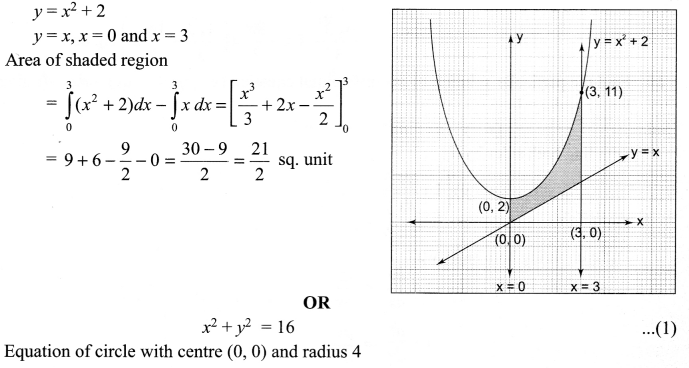
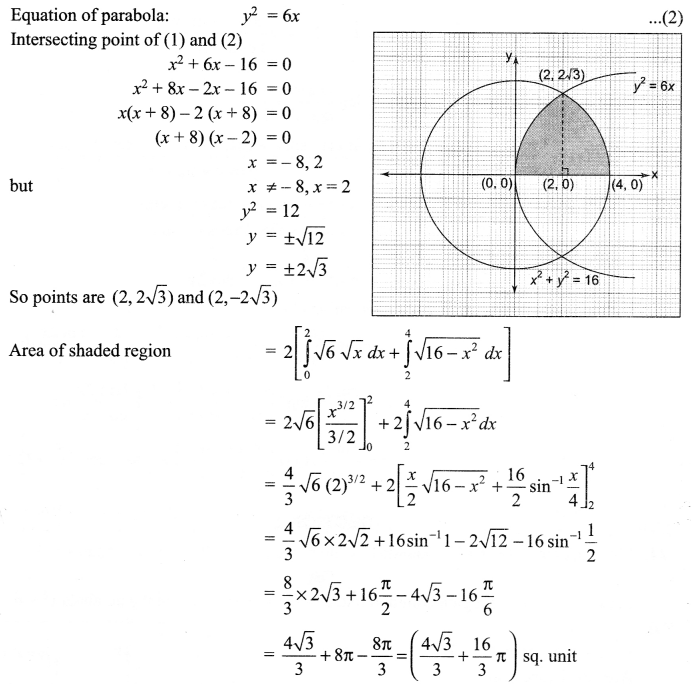
Solution 25.
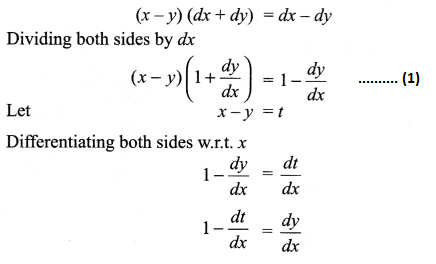
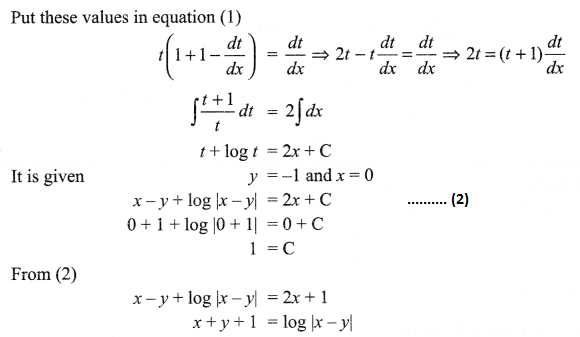
Solution 26.
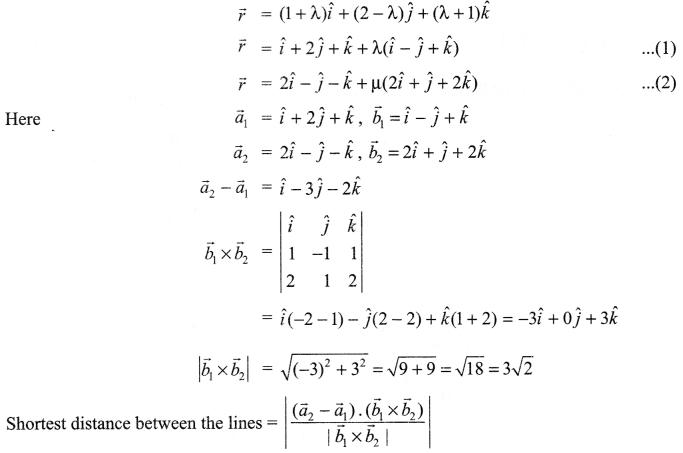
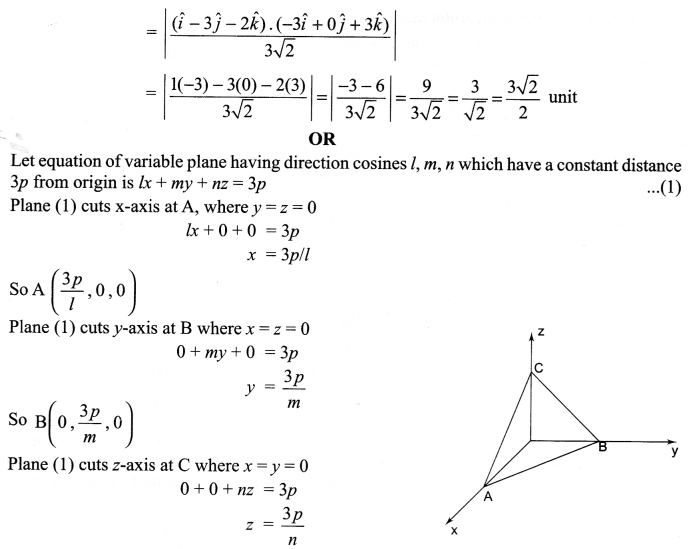
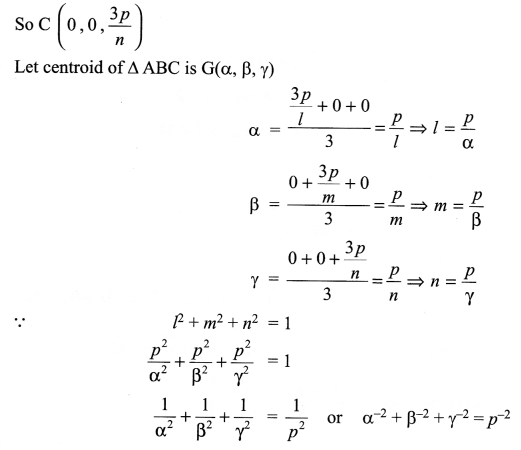
Solution 27.

Solution 28.
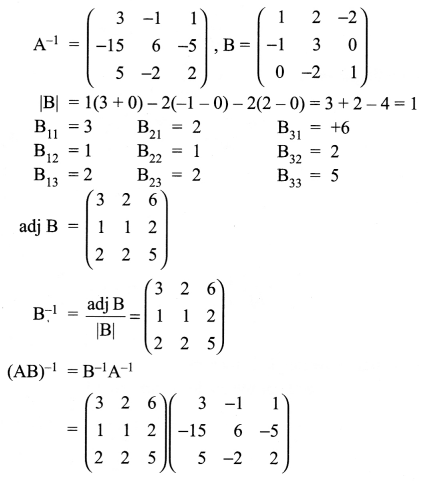
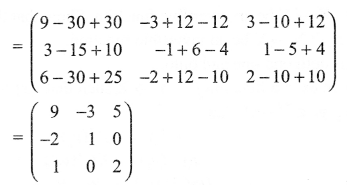
Solution 29.
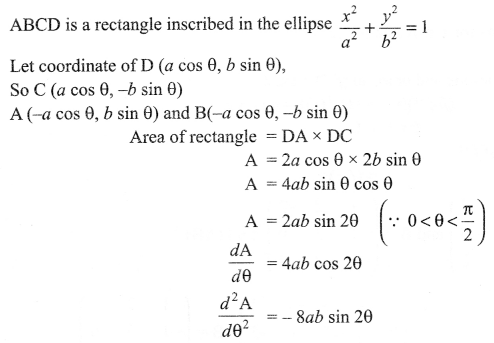
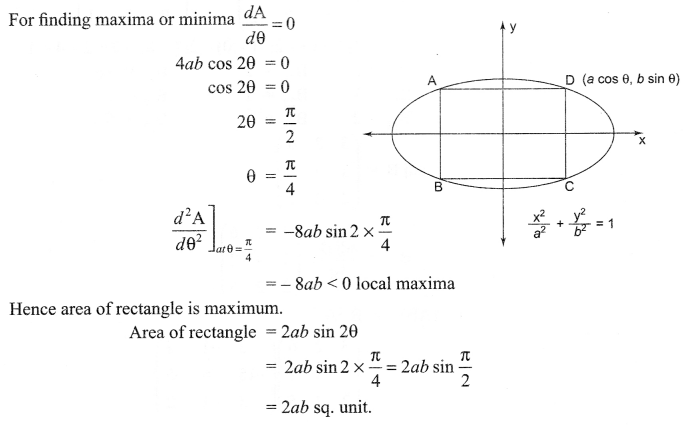
We hope the CBSE Sample Papers for Class 12 Maths Paper 3 help you. If you have any query regarding CBSE Sample Papers for Class 12 Maths Paper 3, drop a comment below and we will get back to you at the earliest.
