Analysing Forces in Equilibrium
The forces acting on an object are in equilibrium when the resultant force acting on the object is equal to zero. An object at rest is in equilibrium.
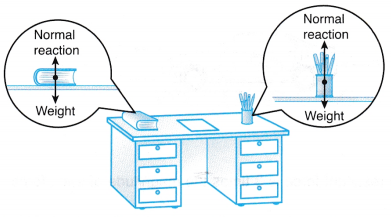Figure shows some objects resting on a table. For the case of the book, the two forces acting on it are its weight and the normal reaction from the table. The two forces are equal in magnitude but act in opposite directions. The resultant force acting on the book is equal to zero. Similarly, the forces acting on the pen stand are also in equilibrium.
Resultant Force
A resultant force is a single force that represents the combined effect of two or more forces by taking into account both the magnitude and the direction of the forces.
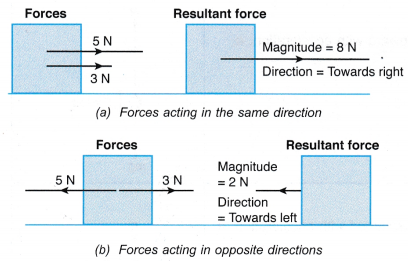Figure shows two examples of a resultant force.
Example 1. A trolley is acted upon by two forces as shown in Figure.
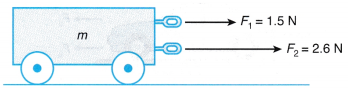Calculate the magnitude and direction of the resultant force.
Solution:
Magnitude of the resultant force = 1.5 + 2.6
= 4.1 N
The direction of the force is the same as the direction of the two forces.
Example 2. Jerak and Jesim made a cart. Their father refuses to let them play outside of the house. Figure shows their father and Jerak pulling the cart.
Calculate the magnitude and direction of the resultant force.
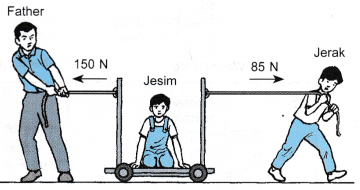
Solution:
The magnitude of the resultant force = 150 – 85 = 65 N
The resultant force is in the same direction as the direction of the force by their father.
Example 3. F1 F2 and F3 are forces with the same magnitude acting on a toy car. Which diagram shows the maximum resultant force acting on the toy car?
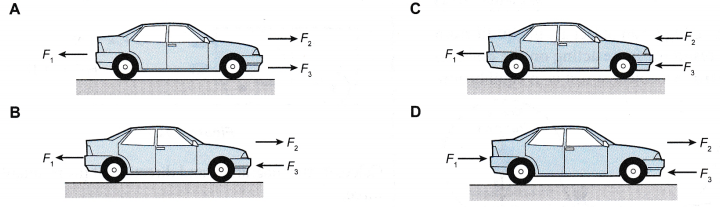
Answer: C
All the forces act in the same direction. The magnitude of the resultant force is 3 times the magnitude of each force.
Applications of Resultant Force
When a person is standing in a lift, her legs act on the floor of the lift. The reaction of the floor acts on her in the opposite direction. The reaction, R, acting on the person can be determined if she stands on a weighing machine.
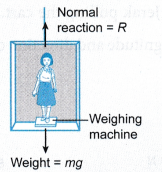 | If the lift is (i) at rest, (ii) moving upward or downward with constant velocity. a = 0 Hence, F = R – mg = 0 Therefore, R = mg |  |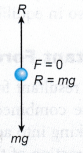
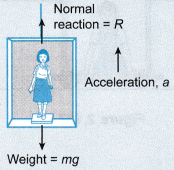 | If the lift is moving upward with acceleration, a, F = ma But, F = R – mg Hence, ma – R – mg R = mg + ma |  |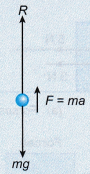
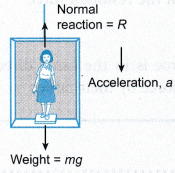 | If the lift is moving downward with acceleration, a, F = ma But, F = mg – R Hence, ma = mg – R R = mg – ma |  |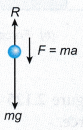
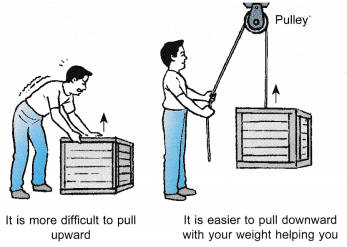
A pulley is very useful for doing work. It can be used to lift loads and change the direction of a force. It is often used in the study of resultant forces.
Example 4. Subra stands on a weighing machine in a lift. If the mass of Subra is 58 kg, determine the reading of the weighing machine when the lift is
(a) stationary
(b) moving upward with a uniform velocity of 2 m s-1
(c) moving upward with a uniform acceleration of 1 m s-2
(d) moving downward with a uniform acceleration of 1 m s-2
(Consider gravitational acceleration, g to be 10 m s-2.)
Solution:
Mass of Subra, m = 58 kg
(a) If the lift is stationary,
The reading of the weighing machine, R = mg
= 58 x 10 = 580 N
(b) If the lift moves upward with a uniform velocity, a = 0,
The reading of the weighing machine, R – mg
= 58 x 10 = 580 N
(c) If the lift is moving upward with a uniform acceleration of 1 m s-2,
The reading of the weighing machine, R = m(g + a)
= 58(10 + 1) = 638 N
(d) If the lift is moving downward with a uniform acceleration of 1 m s-2,
The reading of the weighing machine, R = m(g – a)
= 58(10 – 1) = 522N
Example 5. A 1.5 kg trolley is being pulled by a 0.5 kg weight with the help of a pulley system.
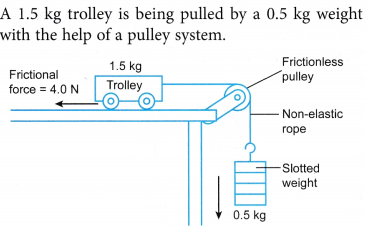
Calculate the acceleration of the trolley. (Take g = 10 m s-2)
Solution:
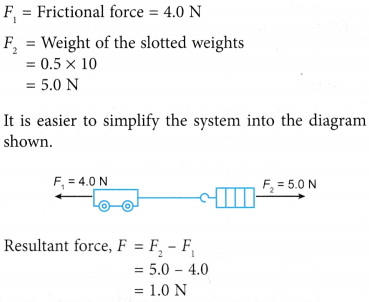
Since both the slotted weights and the trolley are moving together, the total mass of the system, m = mass of the trolley + mass of the slotted weight
Example 6. Figure shows a pulley system with two loads, A and B, connected by a non-elastic rope that passes over a frictionless pulley. (Take g = 10 m s-2)
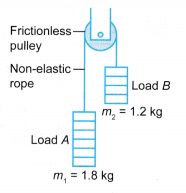
(a) Determine the resultant force, F, of the system and state the direction of the movement of each load.
(b) Calculate the magnitude of the acceleration of load A.
Solution:
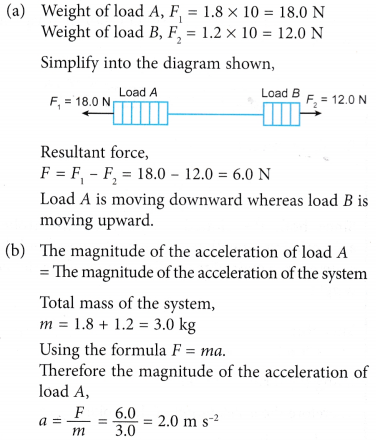
Parallelogram of Forces
The resultant force of two forces acting at a point can be determined by using the parallelogram of forces method.
Figure shows the use of the parallelogram of forces to determine the resultant force, F of F1 and F2.
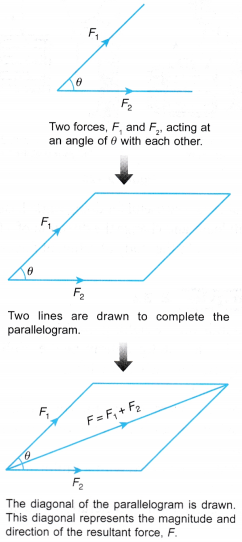
Example 7. Figure shows a boat being pulled by two forces with a magnitude of 300 N and 400 N respectively. The angle between the forces is 60°.
Determine the magnitude and direction of the resultant force using the parallelogram of forces method.
Solution:
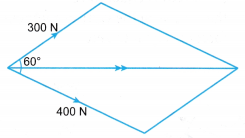Using the scale of 1 cm representing 100 N of force, the magnitude and direction of the resultant force is shown in Figure.
Magnitude of the resultant force = 6.1 x 100 = 610 N
The direction of the force is as shown in Figure.
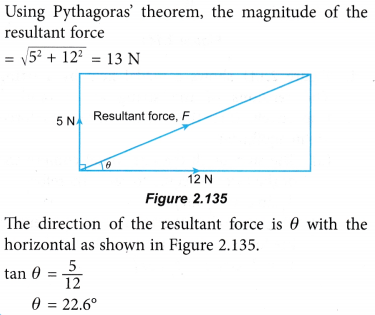
Example 8. Two forces of magnitude 5 N and 12 N act at a point respectively as shown in Figure. The forces are perpendicular to each other.
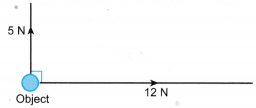What is the magnitude and direction of the resultant force?
Solution:
Resolution of Forces
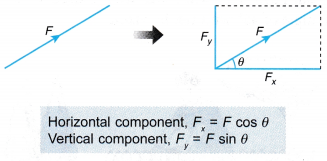A single force can be resolved into two perpendicular components.
Figure shows how a force, F can be resolved into two perpendicular components, Fx and Fy.
Example 9. A man pulls a sack of soil with a force of 125 N at an angle of 40° with the floor.
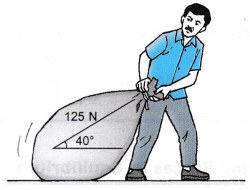Determine the horizontal and vertical components of the force.
Solution:
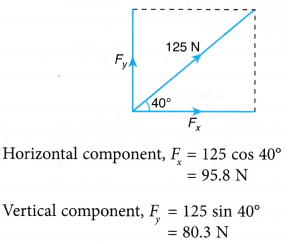
Example 10. A man pushes a lawnmower with a force of 85 N at an angle of 45° with the horizontal.
Calculate
(a) the horizontal component of the force that causes the lawnmower to move forward,
(b) the vertical component of the force that presses on the lawn.
Solution:
Analysing Forces in Equilibrium
- Figure shows a cat standing on an awning. Since the cat is at rest, the forces acting on the cat, its weight, normal reaction and friction, are in equilibrium. The resultant force is zero.
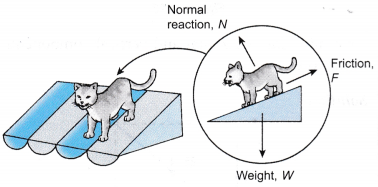
- When the forces acting on an object are in equilibrium, the resultant force acting on the object is equal to zero. If the forces are resolved into horizontal and vertical components respectively, then
(a) the sum of all the horizontal components of the forces is equal to zero,
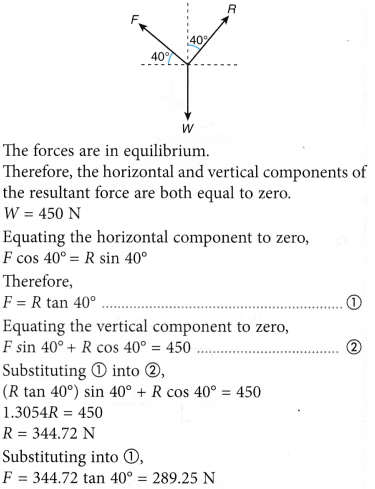
(b) the sum of all the vertical components of the forces is equal to zero. - Figure shows a shoe resting on an inclined surface. The forces acting on the shoe are in equilibrium.
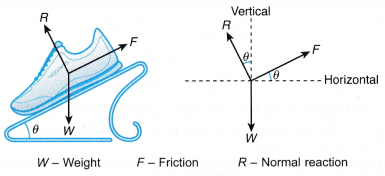(a) The sum of all the horizontal components of the forces is equal to zero. Therefore,
F cos θ = R sin θ.
(b) The sum of all the vertical components of the forces is equal to zero. Therefore,
W = F sin θ + R cos θ. - Figure shows a medal held by a string. The tensions of the string are T1 and T2 respectively. Since the medal is at rest, the forces are in equilibrium.
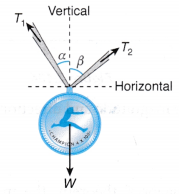(a) The sum of all the horizontal components of the forces is equal to zero. Therefore,
T1 sin α = T2 sin β
(b) The sum of all the vertical components of the forces is equal to zero. Therefore,
W = T1 cos α + T2 cos β
Example 11. Figure shows a traffic light weighing 200 N being held by two identical cables at an angle of 60° with the horizontal beam.
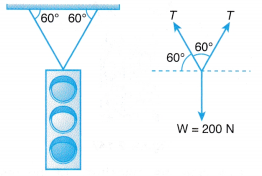Calculate the tension of each cable.
Solution:
Let T be the tension of each cable.
The forces are in equilibrium, the resultant force is equal to zero.
Resolving vertically,
T sin 60° + T sin 60° = W
2T sin 60° = 200
T = 115.5 N
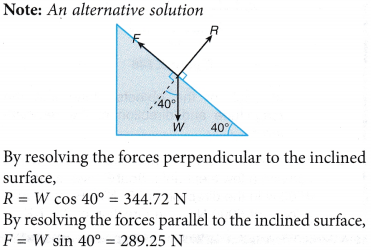
Example 12. A boy weighing 450 N sits on a surface inclined at an angle of 40° with the horizontal floor.
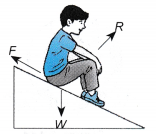Calculate the normal reaction, R and the frictional force, F acting on him.
Solution:
