Ambiguous Case Law of Sines
In Geometry, we found that we could prove two triangles congruent using:
SAS – Side, Angle, Side
ASA – Angle, Side, Angle
AAS – Angle, Angle, Side
SSS – Side, Side, Side
HL – Hypotenuse Leg for Right Triangles.
We also discovered that SSA did not work to prove triangles congruent.
We politely called it the Donkey Theorem ; – )
By definition, the word ambiguous means open to two or more interpretations.
Such is the case for certain solutions when working with the Law of Sines.
• If you are given two angles and one side (ASA or AAS),
the Law of Sines will nicely provide you with ONE solution
for a missing side.
• Unfortunately, the Law of Sines has a problem dealing with SSA.
If you are given two sides and one angle (where you
must find an angle), the Law of Sines could possibly provide you
with one or more solutions, or even no solution.
Before we investigate this situation, there are a few facts we need to remember.
Facts we need to remember:
1. In a triangle, the sum of the interior angles is 180º.
2. No triangles can have two obtuse angles.
3. The sine function has a range of .
4. If sin θ= positive decimal < 1, the θ can lie in the first quadrant (acute <) or in the second quadrant (obtuse <).
Let’s look at some cases. In each example, decide whether the given information points to the existence of one triangle, two triangles or no triangles.
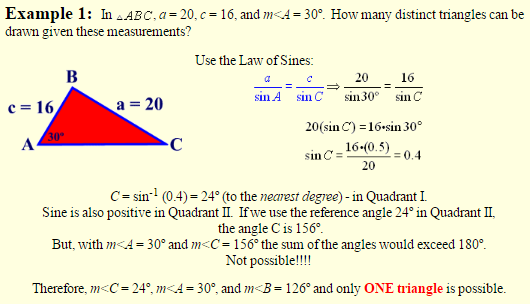
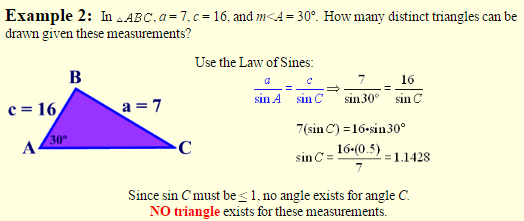
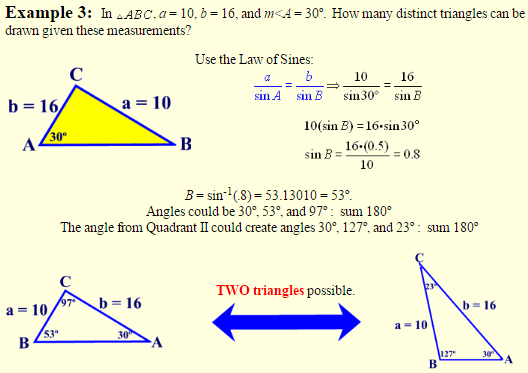
This example is the Ambiguous Case. The information given is the postulate SSA (or ASS, the Donkey Theorem), but the two triangles that were created are clearly not congruent. We have two triangles with two sides and the non-included angle congruent, but the triangles are not congruent to each other.
