Algebra 1 Common Core Answers Student Edition Grade 8 – 9 Chapter 12 Data Analysis and Probability Exercise 12.3
Algebra 1 Common Core Solutions
Chapter 12 Data Analysis and Probability Exercise 12.3 1CB





Therefore, clearly from the above explanation data set 2 is having a greater standard deviation.
Chapter 12 Data Analysis and Probability Exercise 12.3 1LC


The mode is the data item that occurs the most times The data values 33 occur two times in the given data set (1), thus the mode is 33 To explain which measure best describe the data: Observe the given data set (1) for an outlier We see that there is an outlier 1. since an outlier is the data value that is much greater or less than the other values in the data set- If the data set has an outlier then use median to describe the middle of a set of data Thus, the median is the best measure to describe the data
Chapter 12 Data Analysis and Probability Exercise 12.3 2CB





Therefore, clearly from the above explanation data set 2 is having a greater standard deviation.
Chapter 12 Data Analysis and Probability Exercise 12.3 2LC

To find the median, first arrange the data values in increasing order
The number of data values given in equation (2). are evenS Hence its median is the mean of the two middle data values
8.2 8.5 8.8 9 9.3 …… (2)
The number of data values given in equation (2), are odd. Hence its median is the middle data values The middle value is 8.8
Mode is the data item that occurs the most times
Here the data given in (1) have no data items which occur more than once Thus the data set has no mode To explain which measure best describe the data:
Check the given data set (1) for an outlier. we see that there is no outlier. in the data set If the data set has no outlier then use mean to describe the middle of a set of data Thus, the Mean is the best measure to describe the data
Chapter 12 Data Analysis and Probability Exercise 12.3 3CB





Therefore, clearly from the above explanation data set i and data set 2 is having equal standard deviation.
Chapter 12 Data Analysis and Probability Exercise 12.3 3LC


Chapter 12 Data Analysis and Probability Exercise 12.3 4CB





Therefore, clearly from the above explanation data set i and data set 2 is having equal standard deviation.
Chapter 12 Data Analysis and Probability Exercise 12.3 4LC
The mean. median. and mode. all are describe the data set by finding a representative or a single value (in case of mode single. multiple or no mode) to measure of central tendency
The mean can be affected or influenced by an outlier Since an outlier is a data value that is much greater or less than the other value, therefore mean can be overstate or understate by outlier in the measure of central tendency.
The median is the middle value of the data items in the set of data, so if we arranged the data in order the outlier is either last of first value in the data set Thus ¡t is not influenced by an outlier The mode is the most commonly occurring piece of data so it is not influenced by the outlier
Chapter 12 Data Analysis and Probability Exercise 12.3 5LC
Consider the following data.
2 10 8 3 Find the range of the above data
Range: The range of a set of data is the difference between the greatest and the least data values The greatest and the least values of the data given in (1) are 10 and 2 respectively.
Range =10—2
=8
Therefore, the student who said the range 8 is correct.
Chapter 12 Data Analysis and Probability Exercise 12.3 6LC

Chapter 12 Data Analysis and Probability Exercise 12.3 7E


Mode is the data item that occurs the most times The data values 10 occur two times in the given data set(1). thus the mode is 10
To explain which measure best describe the data:
Check the given data set (1) for an outlier We see that there is no outlier. in the data set lithe data set has no outlier then use mean to describe the middle of a set of data Thus, the Mean is the best measure to describe the data
Chapter 12 Data Analysis and Probability Exercise 12.3 8E

To find the median, first arrange the data values in increasing order
96 98 98 99 134………… (2)
The number of data values given in equation (2). are odd. Hence its median is the middle data values.
The middle value is 99
Mode is the data item that occurs the most times.
The data values 98 occur two times in the given data set (1), thus the mode is .98
To explain which measure best describe the data:
Check the given data set (1) for an outlier We see that there is an outlier 134. since an outlier is the data value that is much greater or less than the other values in the data set- If the data set has an outlier then use median to describe the middle of a set of data Thus, the median is the best measure to describe the data
Chapter 12 Data Analysis and Probability Exercise 12.3 9E


Mode is the data item that occurs the most times Here the data given in (1) have no data items which occur more than once. Thus the data set has no mode
To explain which measure best describe the data:
Check the given data set (1) for an outlier We see that there is an outlier 120. since an outlier is the data value that is much greater or less than the other values in the data set. If the data set has an outlier then use median to describe the middle of a set of data. Thus, the median is the best measure to describe the data.
Chapter 12 Data Analysis and Probability Exercise 12.3 10E


Mode is the data item that occurs the most times. The data item 15 occur four times in the given data set (1). thus the mode is 15 To explain which measure best describe the data: we check the given data set (1) for an outlier We see that there is no outlier. in the data set. If the data set has no outlier then use mean to describe the middle of a set of data. Thus, the Mean is the best measure to describe the data.
Chapter 12 Data Analysis and Probability Exercise 12.3 11E

Chapter 12 Data Analysis and Probability Exercise 12.3 12E

Chapter 12 Data Analysis and Probability Exercise 12.3 13E

Chapter 12 Data Analysis and Probability Exercise 12.3 14E

Chapter 12 Data Analysis and Probability Exercise 12.3 15E


Chapter 12 Data Analysis and Probability Exercise 12.3 16E


Chapter 12 Data Analysis and Probability Exercise 12.3 17E


Chapter 12 Data Analysis and Probability Exercise 12.3 18E


Chapter 12 Data Analysis and Probability Exercise 12.3 19E


Chapter 12 Data Analysis and Probability Exercise 12.3 20E

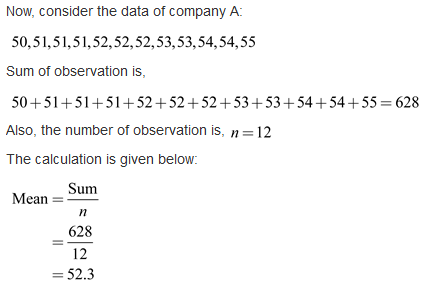

![]()


Chapter 12 Data Analysis and Probability Exercise 12.3 21E






Chapter 12 Data Analysis and Probability Exercise 12.3 22E
Consider that the mean of a data set is 7.8, the mode is 6.6, and the median is 6.8. Find the least possible number of data value in the set. The least possible number of the data set is 6.6. From the above three value we see that the value of mode 6.6 is less than the mean and median values. Now, the mean is defined as the average of the data set- Therefore the average of data set cannot be the least number of the set since mode is less than mean are given Also the median is the middle value in the data set. when data values are arranged in order If the numbers of data values are even in the data set, then the median of an even number of data values is the mean of the two middle data values. Therefore, the median is not least number of data set since mode is less than median are given. Thus, mode is the least number of data set which is .6.6
Chapter 12 Data Analysis and Probability Exercise 12.3 23E





Now, for finding the mode, manufacturing plant A is having mode 5•4 but manufacturing plant B is not having any mode.
Chapter 12 Data Analysis and Probability Exercise 12.3 24E




Chapter 12 Data Analysis and Probability Exercise 12.3 25E
If the same amount is subtracted from each value of the data set, then the mean, median, mode all three are decrease with same amount which was subtracted to its data item. Because all three are the measure of central tendency of data set, means all are represent a data set by its representative. But the range is not affected, since range is the difference of maximum and minimum data valua So if we subtracted the same amount in its maximum and minimum data value then both are decrease in same amount.
Let you subtract the same number d in each items of the set and the set has total n items. Then the sum of the value of the set is decreases by nd. You divided by n to the value nd. since n is the total number to find the mean then mean is decrease by d. The median, since the middle number is decrease by dtherefore the median is also decrease by d. For the mode, since the mode is the most occurring term, so fl each term is decrease by d then mode is also decrease by d. But the range is not decrease.
Range = (hieghest — d) — ( lowest — d) Simplify.
= hieghest — d — lowest + d
= hieghest — lowest
This shows the range is not affected
Chapter 12 Data Analysis and Probability Exercise 12.3 26E
If we divided by same nonzero amount to each value of the data set. then the mean. median. mode all three are affected. The mean, median. mode, and range are decrease with same ratio to its previous value. Since the division of nonzero number decrease the value of data set in the multiple of dividend. Thus the mean. median, mode, and range all are decrease with the multiple of dividend.

Chapter 12 Data Analysis and Probability Exercise 12.3 27E



Chapter 12 Data Analysis and Probability Exercise 12.3 28E

Chapter 12 Data Analysis and Probability Exercise 12.3 29E

Chapter 12 Data Analysis and Probability Exercise 12.3 30E



Chapter 12 Data Analysis and Probability Exercise 12.3 31E

Chapter 12 Data Analysis and Probability Exercise 12.3 32E

Chapter 12 Data Analysis and Probability Exercise 12.3 33E


Chapter 12 Data Analysis and Probability Exercise 12.3 34E
Two points are 2.5 in apart on a map with a scale on 1 in: 1oo m Find the actual locations represented by the points on the mapS The two point are 2.5 in. apart. and the 1 in 100 m therefore to find the actual location that represent the points on the map is obtained by multiply 2.5 in. with 100 m
Distance between two points = 2.5 x 100
= 250 m.
Thus, the two points are 250 m apart on map
Chapter 12 Data Analysis and Probability Exercise 12.3 35E
Consider the height of the professional basketball players:
85 in. 82 in 83 in. 84 in. 80 in 82 in. 86 in. 85 in. 83 in. 84 in. 81 in. 82 in………………….. (1)
Sketch the Histogram of the above data
A histogram is a graph that can be display data from a frequency table. A histogram has one bar for each interval and the height of each bar show the frequency of the data in that interval.


Chapter 12 Data Analysis and Probability Exercise 12.3 36E
Consider the numbers of cars.
53 84 22 38 41 27 25 12 17 27 33 41 60 73 62 59 43…………. (1)
Sketch the histogram of the above data
A histogram is a graph that can be display data from a frequency tabla A histogram has one bar for each interval and the height of each bar show the frequency of the data in that interval


Chapter 12 Data Analysis and Probability Exercise 12.3 37E
Consider the set of points:
(O.1),(1,3),(2,9),(3,27),(4,8I) . (1)
Graph each set of points and tells which model linear quadratic. or exponential is the most appropriate.
Consider the set of points in the xy-plane. Let the x-axis is mark 1 unit length and the y-axis is mark with 9 units. To graph the point (o, 1) in the xy-plane we take x-axis as 0 lengths and y-axis as 1 unit and then marked the corresponding points. To graph the point (1.3) we take x-axis as 1 unit length and y-axis height as 3 units and then marked the corresponding points. Similarly we can graph the point (2,9) by taking x-axis as 2 units length and y-axis height as 9 units and then marked the corresponding points. As shown below:

Chapter 12 Data Analysis and Probability Exercise 12.3 38E
Consider the set of points:
(6,5),(7,2),(8,-1),(9,-4),(1o,-7) ………………. (1)
Graph each set of points and tells which mode? linear quadratic. or exponential is the most appropriate
Sketch the set of points in (1)the xy-plane. Let the x-axis is mark 1 unit length and the y-axis is mark with 2 units
To graph the point (6,5) in the xy-plane we take x-axis as 6 lengths and y-axis as 5 unit and then marked the corresponding points. To graph the point (7,2) we take x-axis as 7 unit length and y-axis height as 2 units and then marked the corresponding points. Similarly we can graph the point (8.,-1)by taking x-axis asS units length and y-axis height as-1 units and then marked the corresponding points. As shown below:

Chapter 12 Data Analysis and Probability Exercise 12.3 39E
Consider the data set.
0 2 7 10 -1 -4 -11 ….. (1)
Find the median, and range of the data set. To rind the median, check the data values given in (1) are arranged in order. The data values given in (1) are not arranged in order, so we arranged them in increasing order. We get:
-11 -4 -1 0 2 7 10 ………………(2)
The number of data values given in equation (2), are oddS Hence its median is the middle data values The middle value is
Range: The range of a set of data is the difference between the greatest and the least data values. The maximum and the minimum value in the set of data given in (1) are lo and -11 respectively. So the range is
Range= 10-(-11)
=21
Chapter 12 Data Analysis and Probability Exercise 12.3 40E
Consider the following data,
64 16 23 57 14 22………………. (1)
Find the median, and range of the data set. To find the median, check the data values given in (1) are arranged in order The data values given in (1) are not arranged in order, so we arranged them in increasing order We get:
14 16 22 23 57 64 …. (2)


Chapter 12 Data Analysis and Probability Exercise 12.3 41E
Consider the following data,
2.1 3.3 -5.4 0.8 3.5 ………………. (1)
Find the median. and range of the data set
To find the median, check the data values given in (1) are arranged in order The data values given in (1) are not arranged in order so we arranged them in increasing order We get:
-5.4 0.8 2.1 3.3 3.5…………………(2)
The number of data values given in equation (2). are oddS Hence its median is the middle data values.
The middle values is: 2.1
Range: The range of a set of data is the difference between the greatest and the least data values The maximum and the minimum value in the set of data given in (1) are 3.5 and -5.4
respectively. So the range is Range= 3.5-(-5.4)
= 8.9.
