Absolute Value Inequalities
Solving an absolute value inequality problem is similar to solving an absolute value equation.
Start by isolating the absolute value on one side of the inequality symbol, then follow the rules below:
If the symbol is > (or >=) : (or)
If a > 0, then the solutions to |x| > a
are x > a or x < – a.
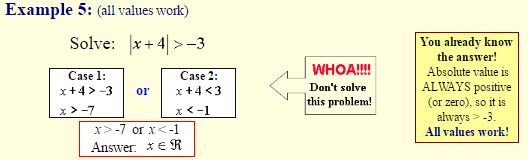
If a < 0, all real numbers will satisfy . |x| > a
Think about it: absolute value is always positive (or zero), so, of course, it is greater than any
negative number.
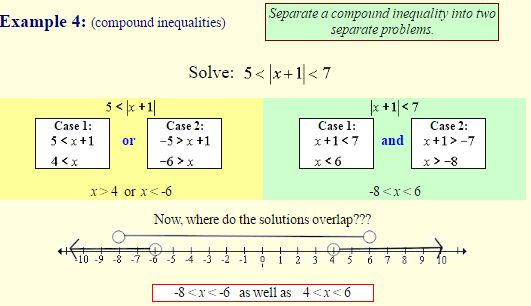
If the symbol is < (or <=) : (and)
If a > 0, then the solutions to |x| < a
are x < a and x > – a.
Also written: – a < x < a.
If a < 0, there is no solution to .|x| < a
Think about it: absolute value is always positive (or zero), so, of course, it cannot be less than a negative number.
Remember:
When working with any absolute value inequality,
you must create two cases.
If <, the connecting word is “and”.
If >, the connecting word is “or”.
To set up the two cases:
x < a
Case 1: Write the problem without the absolute value sign, and solve the inequality.
x > -a
Case 2: Write the problem without the absolute value sign, reverse the inequality, negate the value NOT under the absolute value, and solve the inequality.



Read More:
- Absolute Value
- Absolute Value Equations
- Absolute Value of Complex Numbers
- Integers and Examples
- Fundamental Operations on Integers
- Whole Numbers And Its Properties
- Hints for Remembering the Properties of Real Numbers
- What Are The Four Basic Operations In Mathematics
- Order of Operations and Evaluating Expressions
